On some generalized geometric difference sequence spaces.
Keywords:
Geometric difference, Dual space, Geometric integers, Geometric real numbersAbstract
In this paper we introduce the generalized geometric difference sequence spaces 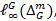

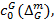 and to prove that these are Banach spaces. Then we prove some inclusion properties. Also we compute their dual spaces.
and to prove that these are Banach spaces. Then we prove some inclusion properties. Also we compute their dual spaces.
References
A. E. Bashirov, E. Misirli, Y. Tandoğdu, A. Özyapici, On modeling with multiplicative differential equations, Appl. Math. J. Chinese Univ. 26 (4), pp. 425-438, (2011).
A. E. Bashirov, E. M. Kurpinar, A. Özyapici, Multiplicative Calculus and its applications, J. Math. Anal. Appl. 337, pp. 36-48, (2008).
A. E. Bashirov, M. Riza, On Complex multiplicative differentiation, TWMS J. App. Eng. Math. 1 (1), pp. 75-85, (2011).
K. Boruah, B. Hazarika, Application of Geometric Calculus in Numerical Analysis and Difference Sequence Spaces, J. Math. Anal. Appl 449 (2), pp. 1265-1285, (2017).
A. F. Çakmak, F.Başar, On Classical sequence spaces and non-Newtonian calculus, J. Inequal. Appl. 2012, Article ID 932734, 12pp., (2012).
R. Çolak, M. Et, On some generalized difference sequence spaces and related matrix transformations, Hokkaido Math. J. 26, pp. 483-492, (1997).
M. Et, Rifat Çolak, On Some Generalized Difference Sequence Spaces, Soochow J. Math. 21 (4), pp. 377-386....
D. J. H. Garling, The - and -duality of sequence spaces, Proc. Camb. Phil. Soc. 63, pp. 963-981, (1967).
M. Grossman, R. Katz, Non-Newtonian Calculus, Lee Press, Piegon Cove, Massachusetts, (1972).
M. Grossman, Bigeometric Calculus: A System with a scale-Free Derivative, Archimedes Foundation, Massachusetts, (1983).
J. Grossman, M. Grossman, R. Katz, The First Systems of Weighted Differential and Integral Calculus}, University of Michigan, (1981).
J. Grossman, Meta-Calculus: Differential and Integral, University of Michigan, (1981).
H. Kizmaz, On Certain Sequence Spaces, Canad. Math. Bull. 24 (2), pp. 169-176, (1981).
G. Köthe, Toplitz, Vector Spaces I, Springer-Verlag, (1969).
G. Köthe, O. Toplitz, Linear Raume mit unendlichen koordinaten und Ring unendlichen Matrizen}, J. F. Reine u. Angew Math. 171, pp. 193-226, (1934).
I. J. Maddox, Infinite Matrices of Operators, Lecture notes in Mathematics, 786, Springer-Verlag, (1980).
D. Stanley, A multiplicative calculus, Primus IX 4, pp. 310-326, (1999).
S. Tekin, F. Başar, Certain Sequence spaces over the non-Newtonian complex field, Abstr. Appl. Anal. 2013(2013) Article ID 739319, 11 pages. 2013. doi: 10.1155/2013/739319.
C. Türkmen, F. Başar, Some Basic Results on the sets of Sequences with Geometric Calculus, Commun. Fac. Fci. Univ. Ank. Series A1. G1(2), pp. 17-34, (2012).
A. Uzer, Multiplicative type Complex Calculus as an alternative to the classical calculus, Comput. Math. Appl. 60, pp. 2725-2737, (2010).
Published
How to Cite
Issue
Section
-
Attribution — You must give appropriate credit, provide a link to the license, and indicate if changes were made. You may do so in any reasonable manner, but not in any way that suggests the licensor endorses you or your use.
- No additional restrictions — You may not apply legal terms or technological measures that legally restrict others from doing anything the license permits.











