Parabolic perturbation in the family z ?1 + 1=wz?
DOI:
https://doi.org/10.4067/S0716-09172002000100001Keywords:
Rational maps, roots of unity, primitives, periodic cycles, hyperbolic components, mapas racionales, raíces de unidad, primitivas, ciclos periódicos, componentes hiperbólicos.Abstract
Consider the family of rational maps
Fd = {z? fw(z) =1+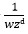 
: w ? C\{0}} (d ? N, d ? 2)
and the hyperbolic component A? = {w : fw has an attracting fixed point}. We prove that if w? ? ?A? is a parabolic parameter with corresponding multiplier a primitive q-th root of unity, q ? 2; then there exists a hyperbolic component Wq; attached to A? at the point w?; which contains w-values for which fw has an attracting periodic cycle of period q.
References
[1] R. Bamon and J.Bobenrieth, ‘The rational maps z?1+1=wz? have no Herman rings’, Proceedings of the American Mathematical Society, (2) 127, pp. 633-636, (1999).
[2] H. Jellouli, ‘Indice holomorphe et multiplicateur’, The Mandelbrot set, theme and variations (ed Tan Lei), London Mathematical Society Lecture Note Series 274 (Cambridge University Press, 2000), pp. 253-264.
[3] M. Lyubich, ‘The dynamics of rational transforms : the topological picture’, Russian Math. Surveys, (4) 41, pp. 43-117, (1986).
[4] J. Milnor, ‘Geometry and dynamics of quadratic rational maps’, Experimental Mathematics, (1) 2 , pp. 37-83, (1993).
[2] H. Jellouli, ‘Indice holomorphe et multiplicateur’, The Mandelbrot set, theme and variations (ed Tan Lei), London Mathematical Society Lecture Note Series 274 (Cambridge University Press, 2000), pp. 253-264.
[3] M. Lyubich, ‘The dynamics of rational transforms : the topological picture’, Russian Math. Surveys, (4) 41, pp. 43-117, (1986).
[4] J. Milnor, ‘Geometry and dynamics of quadratic rational maps’, Experimental Mathematics, (1) 2 , pp. 37-83, (1993).
Published
2017-05-22
How to Cite
[1]
J. Bobenrieth, “Parabolic perturbation in the family z ?1 + 1=wz?”, Proyecciones (Antofagasta, On line), vol. 21, no. 1, pp. 1-7, May 2017.
Issue
Section
Artículos
-
Attribution — You must give appropriate credit, provide a link to the license, and indicate if changes were made. You may do so in any reasonable manner, but not in any way that suggests the licensor endorses you or your use.
- No additional restrictions — You may not apply legal terms or technological measures that legally restrict others from doing anything the license permits.
